IBM Research. 2023. IBM Quantum System One at Shin-Kawasaki (2). Flickr.
<https://www.flickr.com/photos/ibm_research_zurich/52713535856/in/photostream/>
Quantum theory, also known as quantum mechanics, has been highly successful in explaining the behavior of particles at the atomic and subatomic levels. Despite its conceptual challenges and departure from classical intuition, quantum theory has been extensively validated through experimental evidence. Here are some key pieces of evidence supporting the reality of quantum mechanics:
Wave-Particle Duality:
- The wave-particle duality of particles, such as electrons and photons, is a fundamental aspect of quantum theory. Experimental observations, such as the double-slit experiment, demonstrate that particles can exhibit both wave-like and particle-like behaviors, depending on the experimental conditions.
Quantum Entanglement:
- Quantum entanglement is a phenomenon where particles become correlated in such a way that the state of one particle instantaneously influences the state of another, regardless of the distance between them. Numerous experiments, including the violation of Bell inequalities, provide strong evidence for the reality of entanglement.
Quantum Tunneling:
- Quantum tunneling is the phenomenon where particles can pass through energy barriers that classical physics would predict to be impassable. This has been experimentally observed in various contexts, such as in scanning tunneling microscopy and in the behavior of particles in nuclear fusion processes.
Quantum Superposition:
- Quantum superposition allows particles to exist in multiple states simultaneously. Experiments with superconductors and trapped ions have demonstrated the existence of superposition, where particles can exist in multiple energy states at the same time.
Quantum Interference:
- Quantum interference is a phenomenon where the probability amplitudes of different quantum states combine in ways that can enhance or cancel each other out. Experiments, such as those involving interferometers, provide clear evidence of interference patterns consistent with quantum theory.
Quantum Computing:
- The field of quantum computing is based on the principles of quantum mechanics. Quantum computers leverage quantum bits or qubits, which can exist in superposition states, allowing for parallel computation. Ongoing experimental efforts in quantum computing provide practical applications based on quantum principles.
Quantum Cryptography:
- Quantum cryptography exploits quantum mechanical properties for secure communication. Quantum key distribution protocols, such as the BBM92 protocol, use the principles of quantum mechanics to enable secure communication and have been experimentally demonstrated.
These experimental observations and applications provide robust evidence supporting the reality of quantum theory. Quantum mechanics has consistently demonstrated its predictive power and has become an essential framework for understanding the behavior of particles at the quantum level.
Quantum Computers in development

IBM Research. 2023. IBM Quantum System One at Shin-Kawasaki (6). Flickr.
<https://www.flickr.com/photos/ibm_research_zurich/52714013388/in/photostream/>
Quantum computers are a type of computing device that leverage the principles of quantum mechanics to perform certain types of computations more efficiently than classical computers. Unlike classical computers, which use bits to represent information as either 0 or 1, quantum computers use quantum bits or qubits. Qubits can exist in multiple states simultaneously, thanks to the phenomenon known as superposition, enabling parallel processing and potential computational advantages.
Key features and principles of quantum computers include:
Qubits: Qubits are the fundamental units of quantum information. Unlike classical bits that can be in a state of 0 or 1, qubits can exist in a superposition of both states simultaneously. This property allows quantum computers to perform parallel computations on multiple possibilities.
Superposition: Superposition is a quantum principle that enables qubits to exist in multiple states at the same time. This allows quantum computers to process information in parallel, potentially speeding up certain types of computations.
Entanglement: Entanglement is a quantum phenomenon where qubits become correlated with each other in such a way that the state of one qubit is dependent on the state of another, regardless of the distance between them. Entanglement enables efficient information transfer and connectivity in quantum computations.
Quantum Gates: Quantum computers use quantum gates to manipulate qubits. Quantum gates perform operations on qubits to execute quantum algorithms. These gates include operations like Hadamard gates, CNOT gates, and others.
Quantum Parallelism: Quantum computers can explore multiple possibilities simultaneously due to superposition, providing a form of parallelism that classical computers cannot achieve. This can be advantageous for specific types of problem-solving.
Quantum Measurement: Quantum measurement is the process by which a quantum system, such as a qubit, is observed, collapsing its superposition into one of the possible states. Measurement is a crucial step in extracting information from a quantum computer.
Quantum Algorithms: Quantum computers can implement quantum algorithms that take advantage of their unique properties to solve certain problems more efficiently than classical algorithms. Examples include Shor’s algorithm for factoring large numbers and Grover’s algorithm for searching unsorted databases.
Noise and Error Correction: Quantum computers are susceptible to errors due to environmental factors. Implementing error correction is a significant challenge, and researchers are actively working on developing error-corrected quantum computers.
It’s important to note that quantum computers are not intended to replace classical computers but to complement them for specific applications. Quantum computers are expected to excel in areas such as factoring large numbers, optimizing complex systems, and simulating quantum physics, where classical computers face computational bottlenecks. The field of quantum computing is rapidly advancing, with ongoing research and development to overcome technical challenges and enhance the capabilities of quantum computers.
Quantum Mechanics is Foundational to Nuclear Physics

Quantum mechanics plays a crucial role in understanding the behavior of particles at the atomic and subatomic levels, and it is foundational to the field of nuclear physics. While nuclear power plants primarily operate based on principles of nuclear physics, which involve the behavior of atomic nuclei, quantum mechanics underlies many aspects of these processes. Here are some ways in which quantum mechanics is relevant to nuclear power plants:
Nuclear Reactions: Quantum mechanics is essential for describing the interactions and reactions involving atomic nuclei. Nuclear reactions, such as fission (splitting of heavy nuclei) and fusion (combining of light nuclei), are fundamental processes in nuclear power plants. Quantum mechanics helps explain the probabilities and energetics of these reactions.
Quantum Tunneling: Quantum tunneling is a phenomenon where particles can pass through potential barriers, even when classical physics might suggest otherwise. This concept is relevant in understanding certain nuclear reactions and processes, such as alpha decay, where alpha particles tunnel through the potential barrier of the nucleus.
Energy Levels: Quantum mechanics describes the quantization of energy levels in atomic systems. In nuclear physics, this quantization is crucial for understanding the discrete energy levels of atomic nuclei and the transitions between them. These energy level transitions are involved in processes like radioactive decay.
Quantum States of Particles: Quantum mechanics characterizes the quantum states of particles, including those in atomic nuclei. The arrangement of protons and neutrons within a nucleus, as well as the quantum numbers associated with these particles, is critical for determining the stability and properties of nuclear configurations.
Quantum Statistics: Quantum statistics, such as Fermi-Dirac statistics for fermions (e.g., protons and neutrons), are essential for describing the behavior of particles in nuclear matter. These statistics play a role in understanding nuclear structure and interactions.
While quantum mechanics is foundational to nuclear physics, the engineering and practical aspects of nuclear power plants also involve classical physics and thermodynamics. The operation of nuclear reactors involves the control of nuclear reactions, heat generation, and energy transfer, which are described by classical principles. Therefore, while quantum mechanics provides the underlying theoretical framework for nuclear phenomena, the practical application of nuclear energy often involves a combination of quantum and classical physics.
Molecular structure elucidation with charge control

Molecular structure elucidation with charge control can also have implications in the context of quantum mechanics and quantum computers. Here’s how:
Quantum Mechanics:
- Wave Functions and Electronic Structure: In quantum mechanics, the behavior of electrons in a molecule is described by wave functions. These wave functions provide information about the probability distribution of electrons around atomic nuclei, influencing the charge distribution in the molecule.
- Quantum States and Energies: Quantum mechanics helps explain the electronic states and energies of molecules. Computational methods based on quantum mechanics, such as Density Functional Theory (DFT) and ab initio methods, can be used to predict molecular structures and electronic properties accurately.
Quantum Computers:
- Quantum Simulation: Quantum computers have the potential to simulate quantum systems, making them valuable tools for studying molecular structures and properties. They can provide more accurate simulations of quantum states, aiding in the elucidation of complex molecular structures.
- Quantum Algorithms for Chemistry: Quantum computers may offer advantages in solving certain computational problems related to molecular structure elucidation. Algorithms like the Variational Quantum Eigensolver (VQE) are designed to find the electronic structure and ground state energies of molecules efficiently.
Quantum Chemical Calculations:
- Quantum Chemistry Software: Traditional computers, when equipped with specialized software, use quantum chemical calculations based on quantum mechanics to predict molecular structures, energies, and charge distributions.
- Quantum Annealing: Quantum annealers, a type of quantum computing architecture, have been explored for solving optimization problems relevant to molecular structure elucidation. These devices leverage quantum effects to explore potential solutions more efficiently.
In summary, the principles of quantum mechanics play a fundamental role in understanding the electronic structure of molecules. Quantum computers and quantum algorithms offer new possibilities for simulating and solving problems related to molecular structure elucidation, potentially providing more accurate and efficient solutions than classical methods. The intersection of quantum mechanics, computational chemistry, and quantum computing holds promise for advancing our ability to predict and understand molecular properties.
"When a molecule gets charged this also changes the structure of the molecule and in turn the function of the molecule changes." -IBM Research
“Energy conversion and energy transport in living systems relies on charging and discharging molecules. Most important in this aspect is the family of porphyrins, comprising chlorophyll and hemoglobin. The charge transitions of these molecules are essential for life. Charge transitions of molecules play also a crucial rule in organic electronic and organic photovoltaic devices.” -IBM Research
<https://www.flickr.com/photos/ibm_research_zurich/48219647407/>
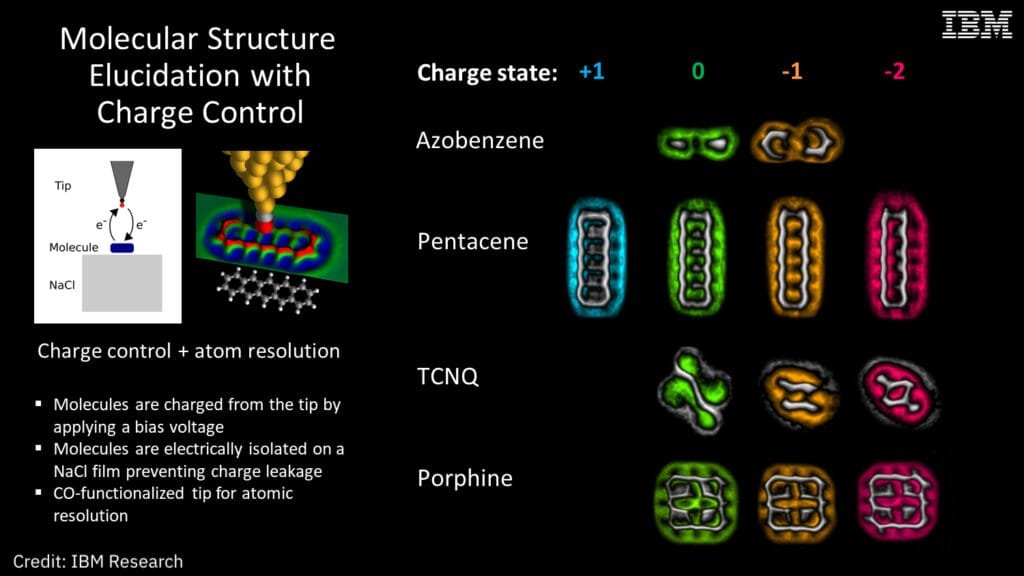
IBM Research. 2019. Explanation Slide. Flickr.
<https://www.flickr.com/photos/ibm_research_zurich/48219647407/>
Molecular structure elucidation with charge control refers to the process of determining the arrangement of atoms in a molecule while also considering the distribution of electric charges within that molecule. This involves techniques and methods used in analytical chemistry to deduce the structural details of a compound, including the positions of atoms and the presence of charged species.
Here are some key components of this process:
Spectroscopic Techniques: Various spectroscopic methods, such as Nuclear Magnetic Resonance (NMR) spectroscopy, Mass Spectrometry (MS), and Infrared (IR) spectroscopy, are employed to gather information about the chemical environment of atoms in a molecule. NMR, for instance, can provide insights into the connectivity of atoms, while MS can give information about the mass and charge distribution.
Charge Determination: Understanding the distribution of charges in a molecule is crucial. This involves identifying ionizable groups, such as acidic or basic functional groups, and determining the formal charges on individual atoms. The presence of charged species significantly influences the chemical behavior and reactivity of a molecule.
Computer Modeling: Computational methods, such as molecular modeling and quantum chemistry calculations, can be used to simulate and predict the molecular structure and charge distribution. These simulations help in interpreting experimental data and refining structural details.
Chemical Analysis: Chemical reactions and derivatization techniques may be employed to modify the molecule in a controlled way, providing additional information about its structure. For example, reactions that selectively react with certain functional groups can help in identifying specific components.
Integration of Data: The results from various analytical techniques are integrated to build a comprehensive understanding of the molecular structure. This involves combining information from NMR, MS, IR, and other methods to create a coherent and consistent structural model.
In summary, molecular structure elucidation with charge control involves a multidisciplinary approach that combines experimental techniques and computational tools to determine the arrangement of atoms in a molecule and understand the distribution of electric charges within that molecular framework. This information is essential for gaining insights into the properties and behavior of chemical compounds.

IBM Research. 2017. Quantum Computer Interior. Flickr.
<https://www.flickr.com/photos/ibm_research_zurich/32390815144/in/photostream/>